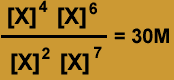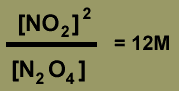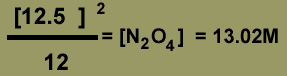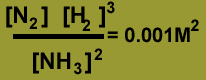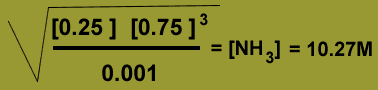Chemistry -equilibrium exercises - calculating reactants and products

Let "X" be the concentration of the species present at equilibrium.
The equilibrium expression therefore looks like

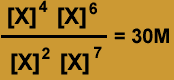
When all cancellations have been done we are left with one "X" expression.
The concentration "X" is = 30M
[X] = 30M

Click to hide the solution

Firstly - find the concentration of dinitrogen tetroxide at equilibrium using the equilibrium expression.

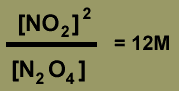
The concentration of nitrogen dioxide is

=>moles / volume = 0.5 / 0.04 = 12.5M

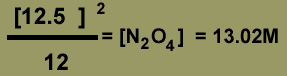
The mole of dinitrogen tetroxide present at equilibrium is given by the expression
mole =concentration X volume
mole =13.02 X 0.04 = 0.52 mole left unreacted at equilibrium.
Since 0.5 mole of nitrogen dioxide was formed then 0.25 mole of dinitrogen tetroxide must have reacted. The total number of mole added to the reaction vessel must be 0.52(mole at equilibrium) + 0.25(mole reacted) =0.77mole.
The mass is = 0.77 X 92=70.84 grams

Click to hide the solution

Firstly - Find the amount in mole of nitrogen gas.
28/28 = 1 mole of nitrogen gas.
The concentration of nitrogen gas is 1mole/4litres =0.25M
The concentration of hydrogen gas is 3mole/4litres=0.75M

Now use the equilibrium expression to find the concentration of ammonia present at equilibrium.

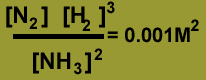
Substituting values give us the expression below

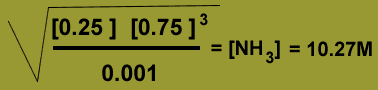
The mole of ammonia = concentration X volume
mole = 10.27 X 4 = 41.1 mole left unreacted at equilibrium.
The amount of mole of ammonia that did react to form 1 mole of nitrogen gas is 2 mole.
Total number of mole = 41.1(mole unreacted) + 2 mole(reacted) = 43.3

Now the mass of ammonia added to the reaction vessel is 43.3 X 17 =698.3g

Click to hide the solution

Calculating reactants and products using "K"


The above reaction takes place in a sealed 3 litre vessel. The reaction is allowed to proceed until equilibrium is reached. The equilibrium constant has a value of 30M at this particular temperature. It was found that the concentration of carbon dioxide, oxygen, water and ethane were identical. Calculate the concentration of each species. Solution

An unknown amount of dinitrogen tetroxide is added to a 40 ml reaction vessel. The compound reacted as per the equation above. The reaction was allowed to come to equilibrium at which point the mass of nitrogen dioxide was measured at 23 grams. If the equilibrium constant at this unspecified temperature is 12M calculate the amount in grams of dinitrogen tetroxide added. Atomc masses N=14, O=16. Solution

An unknown amount of ammonia is placed in a 4 litre sealed container. The above reaction is allowed to reach equilibrium at which point it is found that 28 grams of nitrogen gas is present. If the equilibrium constant at this unspecified temperature has a value of 0.001 calculate the amount, in grams, of ammonia added to the vessel. Solution

Home