Physics-Direction of vectors

Click to hide the solution

A cannon ball is fired skywards with an unknown initial vertical velocity and an initial horizontal velocity of 4 m/s as shown on the right. Ignore air resistance
g=10 m/s/s

a) How long does the ball take to reach its maximum height? (use the formula
d =(1/2)gt²
b) What is the initial vertical velocity of the ball?
c) What is the velocity of the ball just before impact at "B"?
d) How far is point "B" from the launch site?

a) How long does the ball take to reach its maximum height? (use the formula
d =(1/2)gt²

200 = (1/2)10t^{2

=> 40 =}t²
=> 6.32s = t

b) What is the initial vertical velocity of the ball?
v =d/t
=>v = 200/6.32
=>v = 31.65 m/s

c) What is the velocity of the ball just before impact at "B"?
The velocity at impact is equal in magnitude but opposite in sign to the initial velocity.
The magnitude of the initial velocity is obtained by adding the vertical and horizontal velocity vectors. Using the Pythagorean Theorem we obtain a value of 31.90 m/s

d) How far is point "B" from the launch site?
To work out the horizontal distance we must use the horizontal velocity. The ball is in the air for 12.64 seconds. So using hte formula d=vt
distance = 4 m/s X 12.64s = 50.56 m.

Click to hide the solution

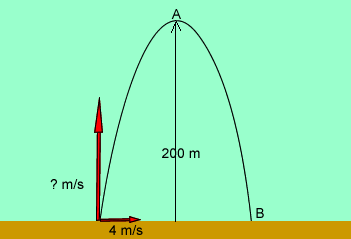
Remember that the horizontal and vertical velocities are independent of each other.

To work out the horizontal velocity we use the formula d/t. Now d = 400m and t = ?
To calculate t we use the formula d = 0.5gt^{2

=> 400 = 0.5 X 10 X t²

=> 80 = t²

=> 8.94s = t

So a vertical distance of 400m must be covered in 8.94s. The vertical velocity
must be

v = 400/8.94 = 44.74 m/s.}

^{To calculate
the horizontal velocity again we use the formula v = d/t.

Now d =600 and t = (2 X 8.94) = 17.88s.

So v = d/t

=>v = 600m/17.88s

=>v = 33.56 m/s}

As the cannon ball passes over the peak its vertical velocity has shrunk to zero. It only has horizontal velocity so it passes at a speed of 33.56 m/s

The cannon ball will strike the target at a speed equivalent to its initial speed. We calculate the initial speed by working out the magnitude of the resultant initial velocity vector.
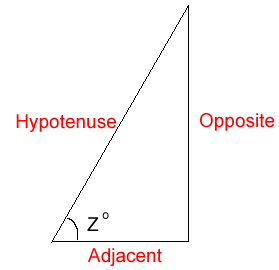
We use Pythagorean Theorem
s² = (hv)² + (vv)² Where s =magnitude of initial velocity vector, hv = initial horizontal velocity and vv = initial vertical velocity.
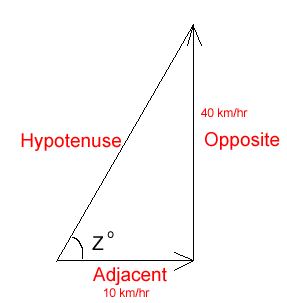
=> s² = (33.56)² + (44.74)^{2

=> s = 55.93 m/s}

Direction
Lets start with the following problem. A plane travels north at 40 km/hr. A 10 km/hr westerly wind is also blowing. Calculate the direction the plane is travelling in. To solve this problem we use trigonometry (in particular sine and cosine)
We will use the following formulae. Since the vectors relating to the velocities of the plane and westerly wind forma right angled triangle we can use the following formulae. Adjacent = hypotenuse X cos ( Z^o ) Opposite = hypotenuse X sin ( Z^o )
Step 1) Using the Pythagorean Theorem we calculate the magnitude of the resultant vector which in this case is represented by the hypotenuse. The magnitude of the resultant vector is 41.23 km/hr. Step 2) Use sin or cos to calculate the angle ( Z^o ) Lets use the sin function and transpose to make it equal to sin( Z^o ). => Opposite/Hypotenuse = sin( Z^o ) => 40/41.23 = 0.97 = sin( Z^o ) => sin^-1 (0.97) = Z^o => 75.97^o = Z^o So the direction is west 75.97^o north. Or north 14.03^o west.
Try the following problems. 1) A plane travels at 30 km/hr north and encounters a 10 km/hr westerly wind. In what direction is the plane travelling? Solution 2) The same plane travels north at 30 km/hr and encounters a 15 km/hr westerly wind . In what direction is the plane travelling? Solution 3) Another plane takes off and travels north at 30 km/hr. and encounter a 15 km/hr easterly. 2) The same plane travels north at 30 km/hr and encounters a 15 km/hr westerly wind . In what direction is the plane travelling? Solution