Rocket activity
Calculating drag.
C Determination of Average Drag Between B and C
Accepting that H + h is the more accurate value for the height reached by the rocket we will use it to work backwards and make an estimate of air resistance(drag) between B and C.
• Subtract your value of
xAB from H + h to get a more accurate value of xBC.
• Use your value of vB (from near top of p.2)and your improved value of xBC to determine the average acceleration between B and C.
This will be done by repeating the calculations on pp 1 and 2 with a value for drag included.
• Note that for the motion from A to B speed increases from 0 to vB , while for the motion from B to C the speed changes from vB to 0. It is therefore a reasonable assumption that the average drag from A to B is the same as that from B to C.
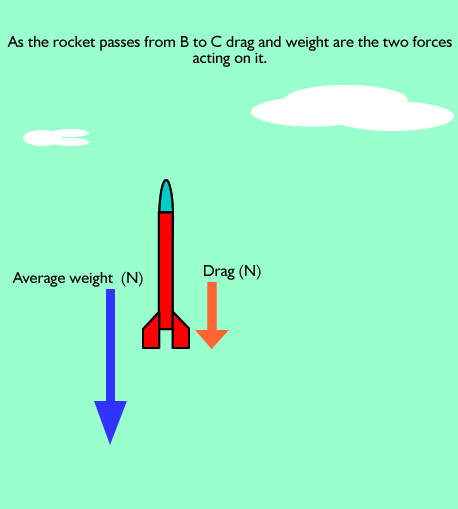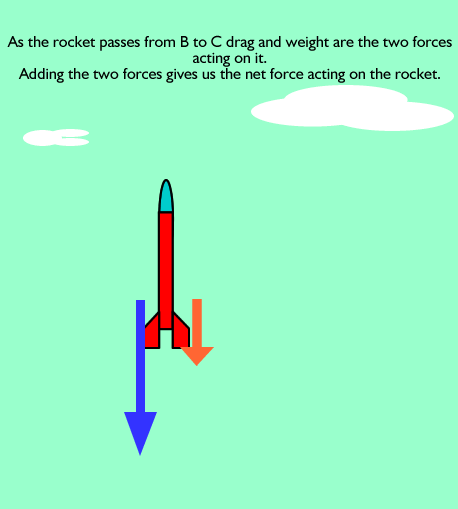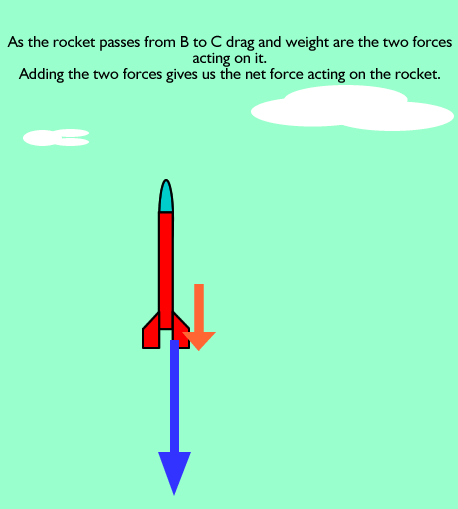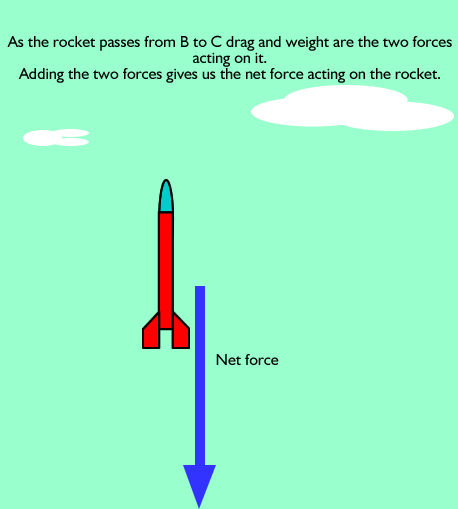
Hide
Maximum height of rocket
= H + h
Mass of rocket before firing = mi
Mass of rocket after fuel is exhausted = mf
Time from A to C (stopwatch) = tAC
Time from A to B = tAB
Time from B to C = tBC
Average drag from B to C = FBC
Vertical component of AB = xAB
Vertical component of BC = xBC
Distance between observers = D
Sight angles of observers = X1 , X2
Speed of rocket at B = vB
Click to hide