Newton's Third Law of Motion

Momentum and collisions
The law of conservation of momentum states that

"When objects that make up an isolated system act upon one another, the total momentum of the system remains constant."
or
The total momentum of an isolated system of interacting objects remains constant."
Collisions are a form of interaction between objects forming an isolated system. Two types of collisions can take place between objects, elastic and inelastic. We will look at the definitions of each later but for the moment it is necessary to stress that, whenever objects collide in the absence of an external force the net momentum of both objects before collision is equal to the net momentum of the objects after the collision.
You may be more familiar with collisions on the sporting field, but not realise the forces acting in each impact.
When objects collide without been permanently deformed or generating heat the collision is said to be elastic. Take the example of two billiard balls colliding. Click to see a 300 Kb video. We see that momentum is transferred completely from one billiard ball to the other. If the balls have the same mass then the speed of the first ball will be the same as that of the second ball.

If the objects differ in mass so will their speeds differ after collision. Let's not forget that the net momentum of both balls should be the same before and after collision. So of the 10 g blue ball travelling at an unknown velocity strikes the stationary 1 g orange ball, the orange ball will surely have to travel at a greater velocity after the collision in order for the net momentum to be the same.

Consider the animation on the right showing an elastic collision between two objects. What is the initial velocity of the blue ball?

Momentum is conserved, so the net momentum before collision equals the net momentum after collision. Since the orange ball is stationary its momentum is 0. The only momentum of the system before collision is due to the moving blue ball. So we can write the expression below.

Mass(blue ball) X velocity(blue ball) = Mass(orange ball) X velocity(orange ball)

=> 0.01 Kg X V(blue ball) = 0.001 Kg X 30 m/s

=> V(blue ball) = (0.001 Kg X 30 m/s) 0.01 Kg

=> V(blue ball) = 3 m/s
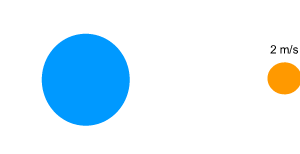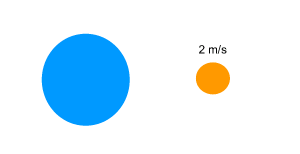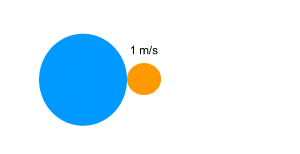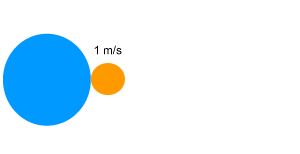
A collision which results in objects becoming distorted and generating heat is known as an inelastic collision. However, the conservation of momentum still applies.
Consider the animation on the right showing an inelastic collision between two objects. If the orange ball has a mass of 6 kilograms can you predict the mass of the blue ball?

Momentum is conserved, so the net momentum before collision equals the net momentum after collision. Since the blue ball is stationary its momentum is 0. The only momentum of the system before collision is due to the moving orange ball. So we can write the expression below.

Mass(coupled balls) X velocity(coupled ball) = Mass(orange ball) X velocity(orange ball)

=> Mass(coupled balls) X 1 m/s = 6Kg X 2m/s

=> Mass(coupled balls)= (6Kg X 2m/s) / 1 m/s

=> Mass(coupled balls)= 12 Kg

=> so the blue ball also has a mass of 6 Kg.

Two players collide with each other as shown on the right. Before the collision the yellow player, with a mas of 120 kg, is stationary, while the other player, also with a mass of 120 kg is approaching from the left. After the collision the total mass of the system is the combined weight of the two player, 240 kg, multiplied by the their speed.

a) What is the momentum of the yellow player just before the collision?

b) The player approaching from the left has a velocity of 4.0 m/s. What is his momentum?

c) After the collision what is the speed of each player?
Solution

Exercises